Understanding Molar Mass: Definition And Calculations For Helium

December 5, 2024 by simbada

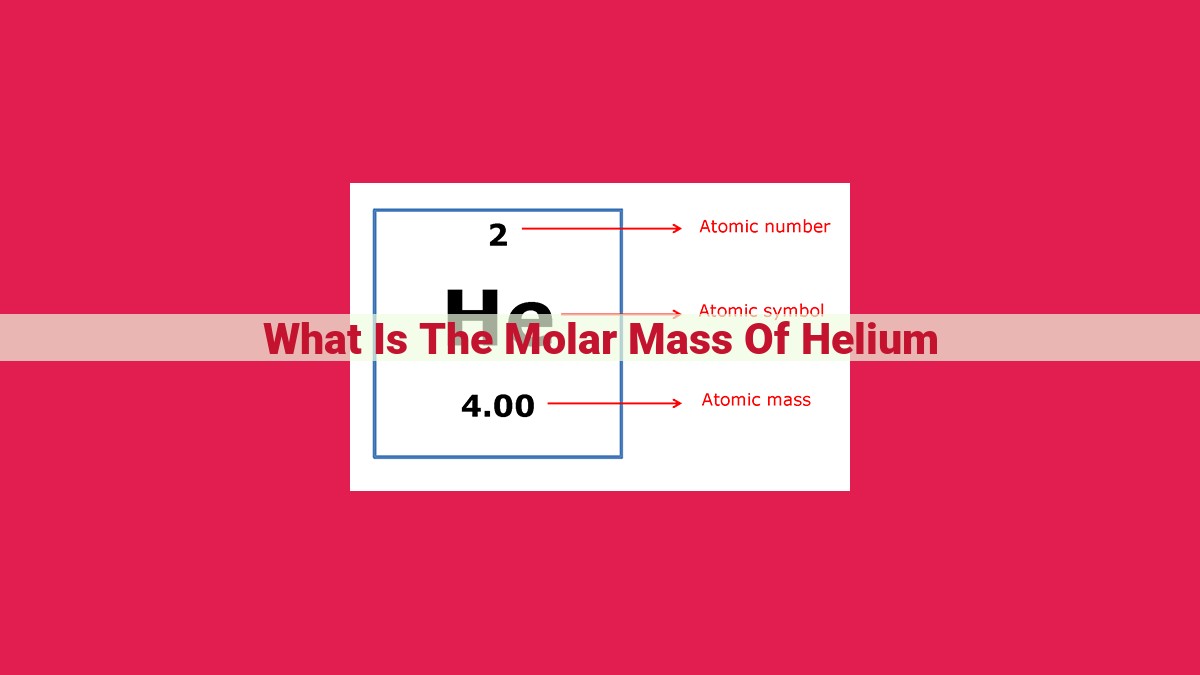
Molar mass is the mass of one mole of a substance, expressed in grams per mole (g/mol). It is calculated by dividing the mass of a specific amount of the substance by the number of moles present. The atomic mass of helium, an element with atomic number 2, is 4.0026 atomic mass units (amu). Using Avogadro's number (6.022 × 10^23 particles per mole) as the conversion factor, helium's molar mass is approximately 4.0026 grams per mole.

The Essence of Molar Mass: Understanding the Mass of a Unit Substance

Molar Mass: The Cornerstone of Stoichiometry

In the realm of chemistry, understanding the mass of substances is crucial for unraveling their properties and interactions. Enter the concept of molar mass, the cornerstone of stoichiometry. Molar mass denotes the mass of one mole of a substance, providing a fundamental measure of the substance's molecular weight.

Molecular Mass, Atomic Mass, and the Power of Spectroscopy

Related to molar mass are other important terms: molecular mass, representing the mass of a single molecule, and atomic mass, the weighted average mass of an element's isotopes. These concepts lay the foundation for understanding the composition of substances. Additionally, techniques like mass spectrometry enable scientists to precisely determine the masses of atoms and molecules, enriching our knowledge of their intricate structures.

Units of Molar Mass: A Guide to Understanding the Basics

In chemistry, the concept of molar mass is fundamental to comprehending the properties and behaviors of substances. It represents the mass of one mole of a compound, providing a critical tool for quantifying and comparing different chemical species. To fully grasp molar mass, it's essential to understand the units in which it's expressed.

The most common unit for molar mass is grams per mole (g/mol). This unit is particularly convenient because it relates directly to the mass of individual molecules or atoms. For instance, the molar mass of water (H2O) is 18 g/mol, indicating that one mole of water molecules weighs 18 grams. Similarly, the molar mass of sodium chloride (NaCl) is 58.44 g/mol, denoting that one mole of sodium chloride weighs 58.44 grams.

Another unit used for molar mass is kilograms per mole (kg/mol). This unit is less frequently encountered but can be useful when dealing with larger quantities of substances. For example, the molar mass of iron (Fe) is 55.85 kg/mol, indicating that one mole of iron atoms weighs 55.85 kilograms.

Understanding the units of molar mass is crucial for accurate calculations in chemistry. By recognizing and correctly using these units, scientists and researchers can determine the masses of substances, relate their compositions to their properties, and develop a deeper understanding of chemical processes.

Unveiling the Essence of Molar Mass: A Journey to Understanding Substance Measurement

Molar mass, the fundamental concept in chemistry, represents the mass of one mole of a substance. A mole, a cornerstone of chemical measurement, embodies an astounding 6.022 x 10^23 entities, whether atoms, molecules, or ions. Understanding molar mass is crucial for comprehending chemical reactions and the quantitative relationships within them.

Formula for Molar Mass: A Guiding Light

The formula for calculating molar mass is an indispensable tool in chemistry:

Molar Mass = (Total Mass of Substance) / (Number of Moles)

This equation serves as a roadmap, guiding chemists to decipher the molar mass of any substance. By determining the total mass of the substance and dividing it by the quantity of moles present, we arrive at the molar mass, a fundamental property that unveils the substance's chemical identity and behavior.

Example: Unveiling the Molar Mass of Helium

Let's embark on an enlightening journey to calculate the molar mass of helium, an element pivotal in various scientific and industrial applications. First, we need to gather information about helium's atomic properties. Each helium atom possesses an atomic number of 2, indicating the presence of two protons in its nucleus. Its mass number, 4, signifies a total of four nucleons (protons + neutrons) within the nucleus.

Helium, like many elements, exists in various isotopic forms. Its most common isotope, helium-4, is composed of two protons, two neutrons, and two electrons. Helium also has a rarer isotope, helium-3, which contains two protons, one neutron, and two electrons.

Now, let's determine the atomic mass of helium. Atomic mass represents the average mass of all helium isotopes, weighted by their natural abundances. Helium-4, with a mass number of 4, accounts for approximately 99.999% of naturally occurring helium. Therefore, the atomic mass of helium is predominantly influenced by helium-4.

To convert the atomic mass from atomic mass units (amu) to grams per mole (g/mol), we employ a conversion factor derived from Avogadro's number:

1 g/mol = 6.022 x 10^23 amu

With this conversion factor in hand, we can calculate the molar mass of helium:

Molar Mass of Helium = (Atomic Mass of Helium) x (Conversion Factor)
= (4 amu) x (6.022 x 10^23 g/mol / amu)
= **4.0026 g/mol**

Unveiling the Molar Mass: A Cornerstone of Chemistry

The molar mass of helium, 4.0026 g/mol, provides a crucial piece of information, enabling chemists to delve deeper into the realm of chemical reactions and quantitative analysis. It represents the mass of 6.022 x 10^23 helium atoms and serves as a gateway to understanding the stoichiometry of reactions involving helium. Armed with this knowledge, scientists can design experiments, predict product yields, and unravel the intricate tapestry of chemical processes.

Atomic Mass of Helium: Understanding the Building Blocks of an Element

In the realm of chemistry, understanding the composition and properties of elements is crucial. Atomic mass plays a pivotal role in this quest, providing scientists with valuable insights into the nature of each element.

Defining Atomic Mass

Atomic mass is the weighted average mass of an element's isotopes. Isotopes are variations of an element that have the same number of protons but different numbers of neutrons. This characteristic influences the mass of each isotope.

Helium: A Case Study

Let's delve into helium, the second element on the periodic table with an atomic number of 2 and a mass number of 4. Helium has two naturally occurring isotopes:

Helium-3: Contains 2 protons and 1 neutron, resulting in a mass of 3 atomic mass units (amu).
Helium-4: Contains 2 protons and 2 neutrons, resulting in a mass of 4 amu.

Calculating Weighted Average Atomic Mass

To determine the atomic mass of helium, we need to consider the relative abundance of each isotope. Helium-4 is the most abundant isotope, accounting for approximately 99.999% of all helium atoms. Therefore, its mass contribution dominates the overall atomic mass calculation.

Using the formula:

Atomic Mass = (Mass of Isotope 1 x Abundance of Isotope 1) + (Mass of Isotope 2 x Abundance of Isotope 2) + ...

We get:

Atomic Mass of Helium = (3 amu x 0.0001%) + (4 amu x 99.999%) = 4.0026 amu

Units Conversion

In chemistry, molar mass is commonly expressed in grams per mole (g/mol). To convert amu to g/mol, we use Avogadro's number (6.022 x 10^23 particles per mole):

1 amu = (1 g/mol) / (6.022 x 10^23 particles/mol)

Therefore, the atomic mass of helium can also be expressed as:

4.0026 amu × (1 g/mol) / (6.022 x 10^23 particles/mol) = 4.0026 g/mol

Conversion Factor between Atomic Mass Units and Grams per Mole

In the world of chemistry, we often encounter the concept of molar mass, which represents the mass of one mole of a substance. Understanding molar mass is critical for various calculations, including stoichiometry and determining the composition of substances. One important aspect related to molar mass is the conversion between atomic mass units (amu) and grams per mole (g/mol).

Avogadro's Number: A Key Player

The key to understanding the conversion lies in Avogadro's number, which is a fundamental constant in chemistry. It represents the number of atoms or molecules present in one mole of any substance, a staggering value of 6.022 × 10^23. This number serves as a bridge between the microscopic world of atoms and the macroscopic world of grams.

The Conversion Factor: A Bridge between Worlds

Using Avogadro's number, we can establish a conversion factor that allows us to convert between amu and g/mol. The conversion factor is:

1 amu = 1.66054 × 10^-24 g

This conversion factor allows us to effortlessly convert from atomic mass units to grams per mole. For instance, if an atom has an atomic mass of 12 amu, we can determine its molar mass in grams per mole by multiplying it by the conversion factor:

12 amu × (1.66054 × 10^-24 g/amu) = 1.992 × 10^-23 g/mol

Calculating the Molar Mass of Helium

In the realm of chemistry, understanding molar mass is crucial for comprehending the composition and properties of substances. In this blog post, we'll embark on a step-by-step journey to calculate the molar mass of helium, a fascinating element that plays a significant role in our universe.

Step 1: Defining Molar Mass

Molar mass is the mass of one mole of a substance. It's like having a precise scale that measures the exact amount of material in a specific unit, known as a mole.

Step 2: Formula for Calculating Molar Mass

The formula for calculating molar mass is simple:

Molar Mass = Mass of one mole of substance / Number of moles

Step 3: Atomic Mass and Avogadro's Number

Helium's atomic mass is the weighted average mass of its isotopes, each with varying numbers of neutrons in their nuclei. Using Avogadro's number (6.022 x 10^23), we can convert the atomic mass from atomic mass units (amu) to grams per mole (g/mol).

Step 4: Calculation

For helium, with an atomic number of 2 and an atomic mass of 4.003 amu, we use the conversion factor:

1 amu = 1.6605 x 10^-24 g

Plugging in the values:

Molar Mass = (4.003 amu) x (1.6605 x 10^-24 g/amu) = 6.646 x 10^-24 g/molecule

Step 5: Converting to Grams per Mole

One mole of any substance contains Avogadro's number of molecules. Therefore, to obtain the molar mass in g/mol, we multiply the mass per molecule by Avogadro's number:

Molar Mass = (6.646 x 10^-24 g/molecule) x (6.022 x 10^23 molecules/mole) = 4.003 g/mol

Thus, the molar mass of helium is 4.003 g/mol. This information is essential for understanding the mass composition of helium in various applications, from balloons to cryogenics.

The Fascinating World of Molar Mass: Unraveling Helium's Secret

In the realm of chemistry, molar mass plays a pivotal role. Simply put, it's the mass of one mole of a substance, providing a fundamental understanding of the atomic and molecular makeup of matter.

Just like every ingredient in a recipe has its own unique weight, each substance has a distinct molar mass. Imagine baking a cake. You need to measure out precise quantities of flour, sugar, and other ingredients based on their corresponding weights. Similarly, in chemistry, molar mass serves as the measuring spoon for substances, helping us determine the exact amount of a particular element or compound we're dealing with.

Units of Molar Mass

Molar mass is typically expressed in grams per mole (g/mol) or kilograms per mole (kg/mol). These units are essential for understanding how much a substance weighs per "mole."

Formula for Molar Mass

Calculating molar mass is straightforward. We simply divide the mass of one mole of a substance by the number of moles. Like solving a simple math problem!

Atomic Mass of Helium

Let's take helium as an example. Helium, with an atomic number of 2, has an atomic mass of approximately 4 atomic mass units (amu). Atomic mass represents the weighted average of an element's different isotopes.

Conversion Factor: AMU to g/mol

Here comes a key conversion factor: 1 amu = 1.6605 x 10^-24 grams. This factor allows us to convert atomic mass units into grams per mole.

Calculating Molar Mass of Helium

Now, let's put all the pieces together to calculate helium's molar mass.

Step 1: Multiply helium's atomic mass (4 amu) by the conversion factor: 4 amu x (1.6605 x 10^-24 grams/amu) = 6.64 x 10^-24 grams
Step 2: Divide the calculated mass by Avogadro's number (6.022 x 10^23 moles/mole): 6.64 x 10^-24 grams / 6.022 x 10^23 moles/mole = 4.0026 x 10^-24 grams/mole

And there we have it! Helium's molar mass is approximately 4.0026 x 10^-24 grams/mole. This tiny but significant value helps us understand how much helium we're working with in various chemical reactions and practical applications.

Related Topics: